Does cooperation in the prisoner’s dilemma unravel after ridiculously many repeated plays?
 Subscribe to Decision Science News by Email (one email per week, easy unsubscribe)
Subscribe to Decision Science News by Email (one email per week, easy unsubscribe)
GUEST POST BY SID SURI: MONTH LONG PRISONER’S DILEMMA
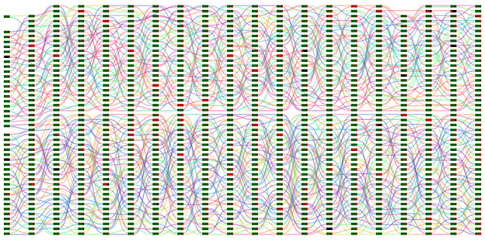
Figure 1: All the games and random rematchings in one out of the twenty sessions we conducted. Each block of green/red shows a game where green represents cooperation and red represents defect. Curves between the games represent how players were randomly rematched.
DSN readers, you are in for a treat this week as Sid Suri, our many-time co-author and colleague at Microsoft Research, is writing a guest post on his repeated prisoner’s dilemma experiment. Many of you heard this experiment summarized in the 2016 Society for Judgment and Decision Making Presidential Address.
Long-run prisoner’s dilemma, a guest post by Sid Suri
Cooperation and more specifically, the Prisoner’s Dilemma, is one of the most studied topics in social science. Yet, despite over 50 years of research and thousands of studies, we still don’t have a good understanding of how people play PD in the long run. The standard theory predicts that, in a finitely repeated game, rational players will use backwards induction to converge on the Nash equilibrium of always defecting. That is, players will cooperate until the last round and defect, and then cooperate until the second to last round and defect and so on until cooperation “unravels” and everyone is defecting on every round. Experimentalists have tried to test this theory by conducting lab experiments where subjects play PD for a few hours. While they might find a little unraveling, these experiments are generally much too short in duration to see how far it goes.
To address this gap in the literature, Andrew Mao, Lili Dworkin, Duncan Watts and I [Sid Suri] conducted an experiment where approximately 100 players each played about 400 ten-round games of PD resulting in almost 400,000 cooperate or defect decisions overall. Subjects were randomly rematched between games and the games were conducted in one-hour sessions, every weekday for a month. (See Figure 1 for a representation of one session.) As these numbers suggest, conducting this experiment online, using a “virtual lab” environment allowed us to skirt some of the logistical limitations of prior lab studies.
Our results showed that cooperation unraveled for the first week and then cooperation levels stabilized after that at above 80%. Furthermore, the reason for this stabilization was due to the roughly 40% of our subject pool who behaved as “resilient cooperators”. These subjects would cooperate until someone else defected on them, and importantly, would not defect on others first, even if they got defected on in a previous game. These resilient cooperators effectively propped up the cooperation levels of the population (see Figure 2). It took us one week of experimentation to just get to the point where cooperation levels stabilized so these findings would have been extremely difficult to see in a lab experiment. We also showed, this time through simulation, that were it not for a sufficient fraction of resilient cooperators, cooperation levels would have unraveled.

Figure 2: Cooperation levels were high and sustained resulting in players earning 84% of the maximum social welfare.
Many prior studies have suggested mechanisms for boosting and sustaining cooperation like punishment, reward, ostracism, and partner selection. Our results suggest that these mechanisms may not be necessary and that a closed population can sustain cooperation on their own provided they have a sufficient fraction of resilient cooperators among them.
REFERENCE
Mao, A., Dworkin, L., Suri, S., & Watts, D. J. (2017). Resilient cooperators stabilize long-run cooperation in the finitely repeated Prisoner’s Dilemma. Nature communications, 8, 13800.


Is this any different than what Thomas Schelling argued for in Micromotives & Macrobehavior?
That is, there may be, depending on the payoffs, a viable coalition of size k such that “though resentful of free-riders, it may be profitable for people to join” & escape the state of nature, page 218.
October 15, 2017 @ 4:01 pm